Drawing of Circles and Pentagon

Objective and Overview

In this unit, students make formal geometric constructions with Polypad's geometry tools while getting a greater insight into geometrical concepts.

In aboriginal times, geometric constructions of figures and lengths were restricted to the utilize of only a straightedge and compasses. Today this tradition tin can exist used to create a serial of puzzle-similar lessons. This unit focuses on the geometric constructions of regular polygons:

• equilateral triangle

• foursquare

• regular pentagon

• regular hexagon

• regular heptagon*

• regular octagon

• regular dodecagon

Warm-up

First with reminding students of the difference betwixt cartoon and constructing a geometric shape. You may want to use the Geometric Constructions - Bones lesson plan before starting this activity.

Ask students to construct an equilateral triangle using only a straightedge and compass. Remind students that they cannot use the ruler to measure lengths or the protractor to measure out the angles. Accept students share their ideas of possible methods. Y'all may as well talk over how circles can exist used to construct an equilateral triangle. Here are two approaches:

• If a certain length is given, place the compass on one end of the segment and ready the compass to the length of the given segment to draw an arc.

• And so keep the width of the compass constant and draw some other arc past placing the compass on the other end of the segment.

• Ii arcs will intersect somewhere above and below the segments. Employ one of the intersections as the third vertex of the equilateral triangle.

• Connect the endpoints of the segment with the intersection point to construct the equilateral triangle.

• If you echo the same steps by cartoon total circles instead of arcs, y'all may over again create an equilateral triangle.

Ask students to measure the angles and the sides of the triangles to prove that it is an equilateral triangle. Talk over with the students why these methods work after each construction.

Main Activity

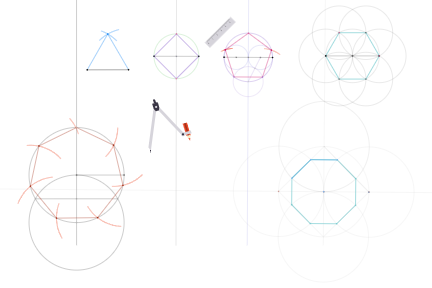
Many regular polygons can likewise be constructed using a straightedge and a compass. Invite students to construct the regular polygons listed in a higher place. Options include presenting them one at a time, sharing them all with students at once, or sharing some with groups. You lot may consider working on these in a few grade periods or over fourth dimension throughout a unit of written report. Below are the solutions for each.

Square

There are different ways to construct a square. For example, you tin can utilise the properties of squares' diagonals to construct one.

The diagonals of the squares have the aforementioned length and are the perpendicular bisectors of each other.

• Construct the perpendicular bisector of the line segment AB.

• Utilise the midpoint of the segment AB to describe a circle.

• Mark and label the points where the circle intersect with the line.

• Connect iv points to construct the square

Y'all may likewise use the bottom toolbar to construct the midpoint, the parallel, and the perpendicular lines directly.

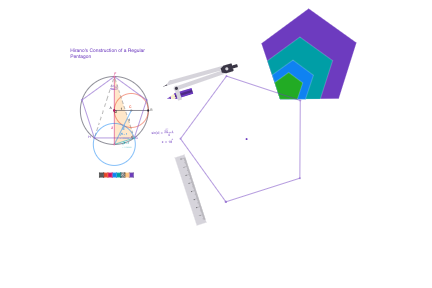
Regular Pentagon

At that place are different means of constructing a regular pentagon. Hirano's construction method of a regular pentagon is one of them. In this method, he uses trigonometry to prove that the construction is authentic.

• Starting time with cartoon a circle with the radius AB, where A is the heart of the circle.

• Mark the midpoint of radius AB with C and depict some other circle with eye C.

• Draw perpendicular line to radius AB through the center A.

• Mark and label the intersection of the circumvolve with the perpendicular line every bit D.

• Connect D and C with a line segment.

• Mark the intersection of the circumvolve with center C and the line segment CD, and label as E.

• Draw another circle with center D and the radius DE.

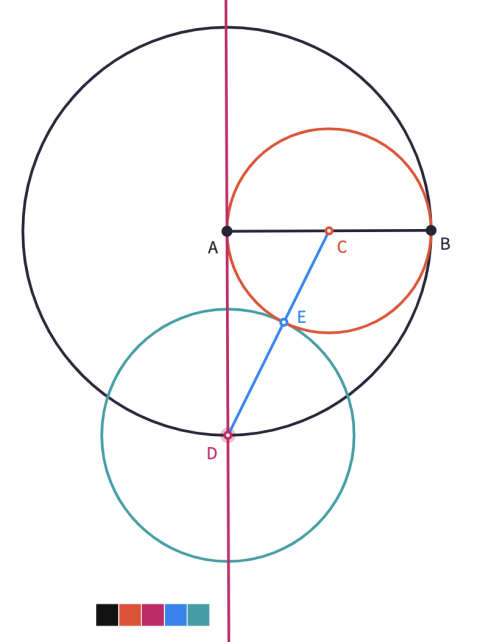
You may want to end hither and ask students the lengths of $\overlinesegment{Air-conditioning}$  , $\overlinesegment{CD}$  , and $\overlinesegment{DE}$  if the length of $\overlinesegment{AB}$  is $2$  units.

Using Pythagoras' Theorem, side lengths of ACD triangle tin can be found. Since $\overlinesegment{Air conditioning}$  and $\overlinesegment{CE}$  are both radii of the circle with the center C, they are congruent. Therefore the side length of $\overlinesegment{DE}$  is $√5-one$  units.

• At present Marker the intersection points of the circles centered A and D , and label them as H and M.

You may demonstrate amalgam the triangle FGD using the custom polygon tool.

$|DF|= 4$  units

Line segment DG is also the radius of the circle with middle D. $|DG|=√five-1$ 

Bending G substends the diameter of the circle, therefore it is 90 degrees. So, nosotros can use the sine value of the bending GFD to find its mensurate.

$sin^{-1}(x)=\frac{\sqrt{5}-i}{iv}$ 

$ten= 18$  $^o$ 

Then, the mensurate of the inscribed bending HFG is 36 degrees that proves the arc measure of the HG as 72 degrees. Since nosotros have proved to split the circumference of the circle five congruent pieces, we can keep and complete the regular pentagon.

• Place the compass on H and arrange its width as HG to describe arcs on the circle. Repeat three more times until you marking v vertices of the pentagon.

Regular Hexagon

A regular hexagon is fabricated upward of half dozen equilateral triangles.

The blossom like shape is used in many areas of art and architecture. If merely the petal-similar arcs are shown it is called a hexafoil. If nineteen flowers come up together, it is usually called the flower of life.

Regular Heptagon

*The regular heptagon is non constructible with compass and straightedge just is constructible with a marked ruler and compass. However, you can construct an approximation for applied use with an mistake of nearly 0.2%.

Regular Octagon

Constructing a regular octagon and dodecagon (12-gon) is quite similar.

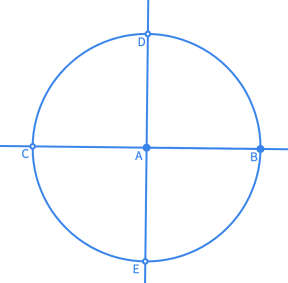
• Start with drawing a circle with eye A.

• Construct the perpendicular diameters equally CB and DE.

• So, connect B, C, D, and East to create a square. (Square Construction)

• Construct iv circles. Each corner of the square should be the center of ane of the circles.

• Construct a bespeak everywhere the circles intersect the square DBEC.

• Connect these points to construct the regular octagon.

• Instead of amalgam points where the circles intersect the square, construct points everywhere that five circles intersect.

• Connect all the points to construct the regular dodecahedron.

Closure

Not all regular polygons are constructible. Polygons with vii, 9, and xi sides are not, for example. Even so, they can be constructed using a marked ruler (neusis constructions).

Enquire students to predict whether a regular 17-gon tin be synthetic or not. After their predictions, yous tin share the story of Gauss, who at the age of fifteen proved that a regular 17-gon tin exist constructed.

Demonstration Polypads For This Lesson

Equilateral Triangle - Polypad - Mathigon

Virtual manipulatives, dynamic geometry, graph plotting, data science and more: explore the ultimate mathematical playground!

Regular Polygons Construction - Polypad - Mathigon

Virtual manipulatives, dynamic geometry, graph plotting, data scientific discipline and more: explore the ultimate mathematical playground!

Regular Pentagon - Polypad - Mathigon

Virtual manipulatives, dynamic geometry, graph plotting, data science and more: explore the ultimate mathematical playground!

mcguinnessgons1995.blogspot.com

Source: https://mathigon.org/task/geometric-constructions-regular-polygons

Share this post